Kinetic Monte Carlo
Microstructure Evolution and Cell Voltage in Li-ions batteries
The kinetic anisotropy of lithium ion adsorption and lithium absorption for LixFePO4 olivine nanocrystals is simulated and reported. The kinetics depend on the orientation of the electrolyte/LixFePO4 interface with respect to the far-field ionic flux. As a consequence of these kinetics and a Li miscibility gap in LixFePO4, the particle geometry and orientation also have an effect on the morphology of the two-phase evolution. These processes accompany the charge and discharge behavior in battery microstructures and a direct influence on battery behavior is suggested. A KMC algorithm based on a cathode particle rigid lattice is used to simulate the kinetics in this system.
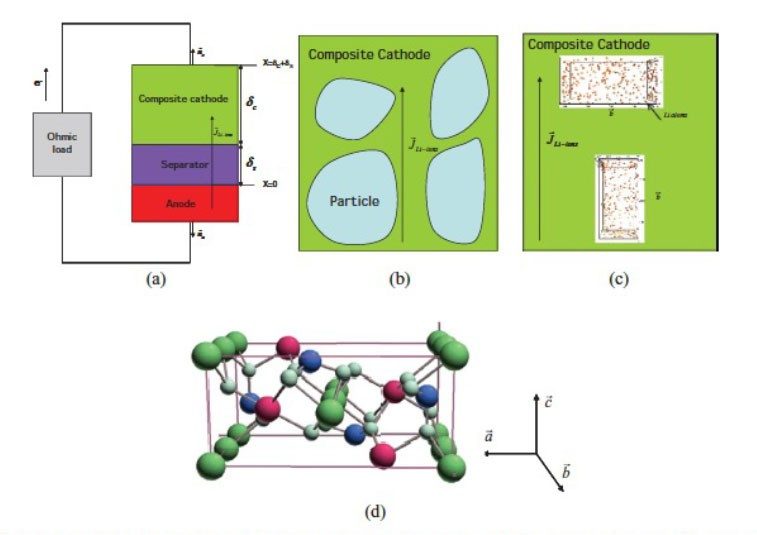
Figure 1. a) Model of the lithium cell used in the simulation. It consists of a lithium negative electrode and a separator cathode region of width ∂s+∂c comprising one particle that acts as an active material. b) Composite cathode used for mesoscopic modeling, it comprises many particles. c) Composite cathode used for atomistic modeling. It comprises one particle. The far-field flux of lithium ion is parallel or perpindicular to the b direction of the cell. d) Lattice model used for the KMC simulation of the Li-FePO4 system. The interstitial sites for lithium atoms are in green, the oxygen atoms are in light blue, the iron atoms are in red, and the phosphate atoms are in dark blue.
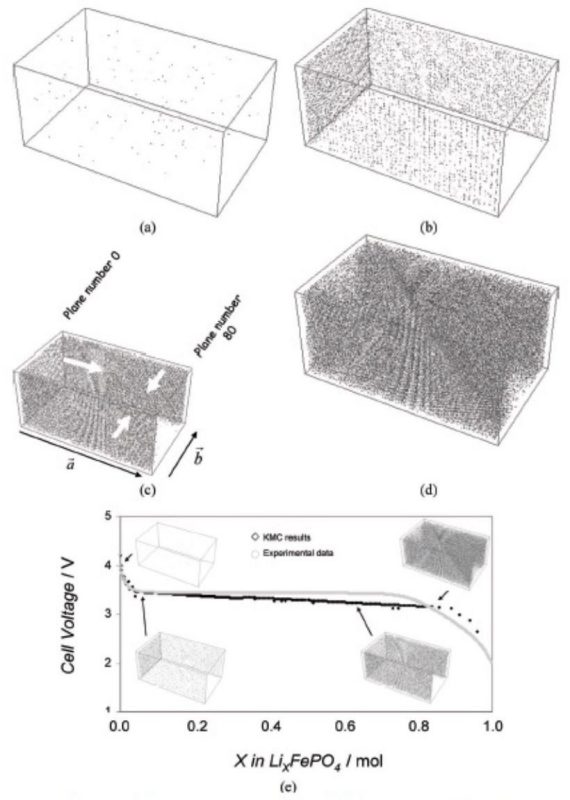
Monte Carlo simulation of the galvanostatic discharge process perfomed at room temperature. The gray points represent the lithium atoms in the active particle. The superficial current density is 0.5 A m-2. The far-field flux of Li ions is perpindicular to the b direction of the cell. Microstructure at: a) 0 s (initial random solid solution), b) 0.0001 s (two Li-enriched phases have formed and joined together), c) 0.00056 s (growth of the Li-enriched phase), d) 10.81 s (the Li-poor phase is almost consumed). e) Cell voltage as a function of Li concentration (mol) in the active material: region 1) decreasing cell potential for Li-insertion into a Li-poor phase; region 2) nearly constant potential after the nucleation of a Li-rich phase; region 3) decreasing cell potential after the Li-poor phase has been consumed by the Li-rich phase.
Nanostructure Ferritic Alloys
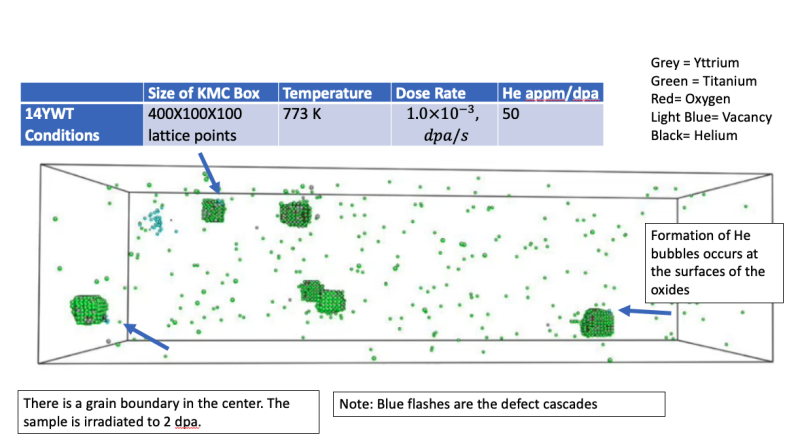
- A newly developed Kinetic Monte Carlo model was constructed to investigate the precipitation behavior of Y-Ti-O oxides along grain boundaries in nanostructured ferritic alloys and the oxide response to neutron irradiation.
- The level of radiation damage simulated reached the long dpa range required for reactor components. Simulations were conducted to investigate the influence of temperature as well as the influence of grain boundary sinks on the resulting oxide characteristics of a 12YWT-like alloy during heat.
- There is good agreement of the oxide characteristics from simulation with literature values and the influence of the grain boundary on precipitation is slight.
- A long-term irradiation simulation at 873K and 10^(-3) dpa/s was taken out to 66 dpa and it was found that the oxides in the vicinity of the grain boundary were more susceptible to dissolution. Additionally, irradiation simulations of a 14YWT-like alloy were conducted to replicate findings from neutron irradiation experiments. The larger oxides in the 14YWT-like alloy simulated in the KMC did not dissolve and showed the same stability as in the experiments.
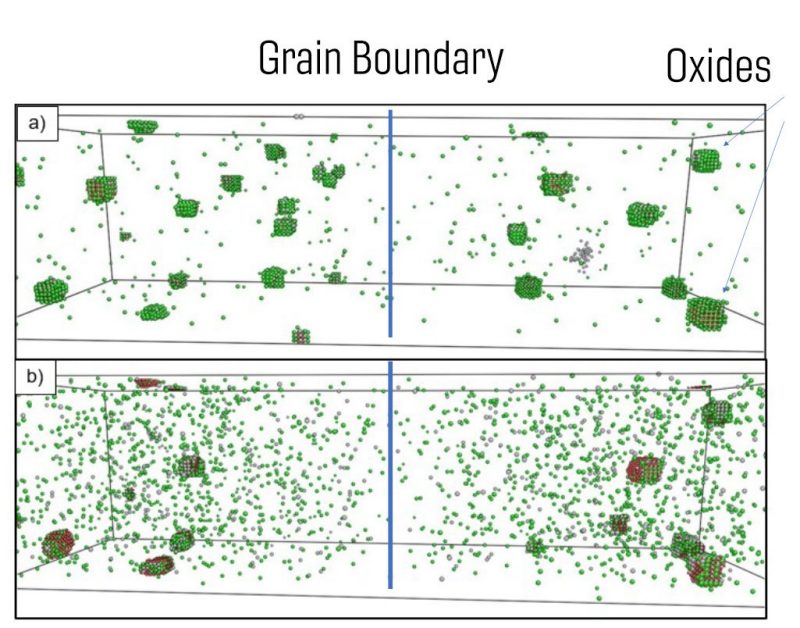
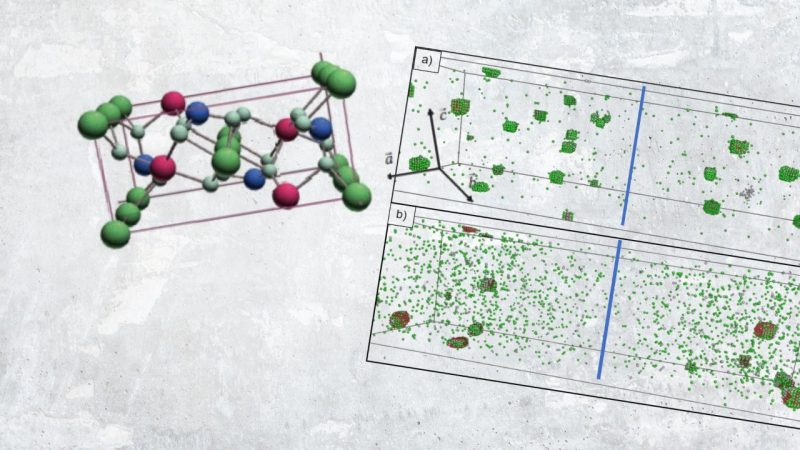
Oxide distributions of the 12YWT NFAs at 1123K a) before neutron irradiation b) After neutron irradiation with total dose 66 dpa at 873K with a dose rate of 10-3 dpa/s. We can observe the Inverse Kinkerdall effect. Green spheres: Ti atoms, Grey spheres: Y atoms, Red spheres: O atoms
Microstructure Evolution of NFAs under neutron irradiation and He implantation